Diagram

[ManhattanPrepLSAT1]

[AlexandraM278]

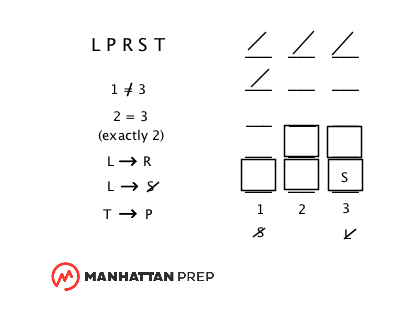
How do we know that each bouquet has 2 types of flowers?

[MitchellL341]

How do we know that each bouquet has 2 types of flowers?

The second and third bouquets must have at least two flowers because they must share two elements in common. It's, thus, guaranteed that 2 and 3 will have at least two spots filled with flowers. All we know based on the stimulus about the first bouquet is that it must have at least one flower, so one spot must be filled.

[GeorgiaV974]

How do we know that S in bouquet 3 is guaranteed to be shared with bouquet 2? Couldn’t there be 2 other types that are shared? So, why is S placed in the 2 guaranteed shared boxes when it could go in any of the 4 spots?

[Laura Damone]

Hi! Sorry for the embarrassing delay in response here. We had a technical difficulty that caused this post to get improperly categorized and lost in the cracks.

In the diagram shown, a box just represents a slot that must be filled. We know 2 and 3 must have 2 flowers in common, so we drew two boxes in each. And we know S is in 3, so we placed S in one of those guaranteed boxes. But that doesn't imply that it's one of the shared flowers. Maybe it is, but maybe it isn't. The diagram doesn't indicate either way.

For a game like this, I think it's better not to diagram in a way that designates certain slots the shared slots because it makes the game play less efficient. When I learn an element must go in group 2 or group 3, I want to be able to place it in there, and then worry about whether it must be shared by the other group later.

Hope this helps!

[AnnaF185]

Hello,

Why are there two slashes for bouquet 1?
I understand that one slash comes from the first rule (1 and 3 cannot have any shared flowers). If either had all five flowers, then it would necessarily share flowers with the other bouquet.
But what account for the second slash, making the maximum 3 flowers?


Update on my thought (8/10/21):

If bouquet 1 were allowed 4 flowers and then actually had 4 flowers, then only 1 flower would be allowed in bouquet 3, the flower that bouquet 1 did not have. Because bouquet 3 requires at least 2 flowers to share with bouquet 2, there is no chance that bouquet 1 could have 4 flowers.

In other words: because bouquet 3 must have at least 2 flowers, bouquet 1 could have at most 3 flowers.

[Misti Duvall]

Hello,

Why are there two slashes for bouquet 1?
I understand that one slash comes from the first rule (1 and 3 cannot have any shared flowers). If either had all five flowers, then it would necessarily share flowers with the other bouquet.
But what account for the second slash, making the maximum 3 flowers?


Update on my thought (8/10/21):

If bouquet 1 were allowed 4 flowers and then actually had 4 flowers, then only 1 flower would be allowed in bouquet 3, the flower that bouquet 1 did not have. Because bouquet 3 requires at least 2 flowers to share with bouquet 2, there is no chance that bouquet 1 could have 4 flowers.

In other words: because bouquet 3 must have at least 2 flowers, bouquet 1 could have at most 3 flowers.

That's right!