GRE Math Riddle: Doubling the Average Rate
You can attend the first session of any of our online or in-person GRE courses absolutely free. Crazy, right? Check out our upcoming courses here.
Here’s a GRE math riddle:
This isn’t exactly a GRE question, but solving it tells you something absolutely crucial about “average rate” questions—which definitely are on the GRE. So take a minute and think about it before you read any further.
…
(I trust you’re doing some good thinking on your own first. It wouldn’t really be a GRE math riddle if I just gave you the answer. ?)
….
(Still thinking? Cool. Keep scrolling when you’re ready to discuss.)
…
Alright, now let’s talk. Like I said, you’d never get something like this on the GRE. But it’s so worth thinking about, I say we play around with it for the rest of this blog entry. First, let’s discuss an obvious answer. An obvious answer that also happens to be a wrong answer: 150 mph.
If you took a direct average of 150 and 50, you’d get 100.
In the good old-fashioned average formula, that looks like:
But, like I said, 150 mph is incorrect for our question. Average speed is not a direct average of two numbers. And it actually follows a slightly more nuanced formula:
Let’s keep playing around with 150 mph to see why it fails. If we plug our given information into the rate formula, we’ll be able to crank out everything else we need.
For the first lap:
Dividing both sides of the equation by 50, you can solve for T. The time it takes for the first lap is 2 hours.
And for the 2nd lap:
We divide both sides by 100 mph and solve for T. The time it takes for the 2nd lap is 100/150… or about .66 hours.
Since each lap was 100 miles, the journey totals 200 miles.
Since the first lap took 2 hours and the second lap took .66 hours, the total time is 2.66 hours.
Not only does 150 mph fail to double our average speed for the whole trip, it actually falls quite a bit short of the overall average of 100 mph we were shooting for. Just for reference, here’s the riddle again:
Doubling the car’s speed for the overall trip requires it to go quite a bit faster on the 2nd lap than it went on the first. Let’s try plugging in a pretty outlandish guess to see what happens. Say the car burns some serious rubber and comes zipping through the 2nd lap at a speed of 400 miles per hour.
The car would make quick work of 100 miles, finishing in just ¼ of an hour.
Plug that in with the time and distance of the first lap, just like we did before:
That’s definitely faster, but it’s still not even close to the 100 miles per hour we were shooting for.
Now let’s cut to the chase. What’s the answer to the GRE math riddle???
The car would need to go infinitely fast for the 2nd lap in order to obtain double its average speed. Even if it was travelling at 5,000 miles per hour, the car wouldn’t quite be able to double its average speed. That’s because it would have to be done with the 2nd lap at the exact moment the lap started. To get an average speed of 100 miles per hour, we’d need this:
Pretty crazy, right? Think about that the next time you’re driving to work.
Actually, don’t. Focus on driving safely. Driving and mathematics are probably a somewhat dangerous pair to combine.
If you’ve got the kind of nerdy friends who are open to it, try asking them this same math riddle. I’m admittedly pretty nerdy, and I tend to hang with a somewhat nerdy crowd, but I’ve found that this question gets some pretty reliable mental fireworks going.
Enjoy! And maybe now you’ve got a good way to truly remember that average rate formula (which definitely is tested on the GRE):
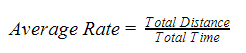
Happy studying! ?
Want more guidance from our GRE gurus? You can attend the first session of any of our online or in-person GRE courses absolutely free! We’re not kidding. Check out our upcoming courses here.
Tom Anderson is a Manhattan Prep instructor based in New York, NY. He has a B.A. in English and a master’s degree in education. Tom has long possessed an understanding of the power of standardized tests in propelling one’s education and career, and he hopes he can help his students see through the intimidating veneer of the GRE. Check out Tom’s upcoming GRE courses here.