GRE Math for People Who Hate Math: Absolute Value
You can attend the first session of any of our online or in-person GRE courses absolutely free. Ready to take the plunge? Check out our upcoming courses here.
Think of an absolute value as a simple machine that looks like this: ||. You put a value into it, and the machine answers a single question for you: how far away from zero was the value that you put in?
The basic operation of the machine is simple. Take any number, put it into the machine, and find out how far from zero that number is. The absolute value of 12, |12|, is equal to 12. The absolute value of -10, |-10|, is equal to 10. That’s because -10 is 10 units away from zero.
It starts to get complicated when the GRE asks you to put things into the machine that are more complex than simple numbers. Imagine that somebody else is operating the machine. She puts values in, but she doesn’t tell you what those values are. All you can see is the answer that the machine gives when it receives those values.
Suppose that the absolute value machine operator – call her Abby, for short – puts a value into the machine, and the machine answers ‘5’. That’s equivalent to saying that |x| = 5, in a GRE problem. What could x be? What did Abby put into the machine? Any value that’s 5 units away from zero. She could’ve inserted either a -5 or a +5, and you would’ve gotten the same result.
What if you put something more complex into the absolute value machine? Suppose that Abby takes an unknown again, but this time, before putting it in the machine, she multiplies it by two and adds one. That is, the expression she puts in is 2x + 1. Then, the machine answers ‘7’. You now know that 2x + 1 is 7 units away from zero.
That allows you to simplify as follows:
|2x + 1| = 7
2x + 1 = 7 OR 2x + 1 = -7
x = 3 OR x = -4
Next, Abby tells you, she’s going to put two values into the machine. She won’t tell you what the values are. But she does tell you that the machine gave the same response to both of them. What do you know about the values?
The two values might or might not be the same, but they’re definitely equally far away from zero:
This scenario is analogous to the equation |x| = |y|. You don’t know what x is, and you don’t know what y is. You don’t know whether the two variables are equal, and you don’t know whether they’re positive or negative. However, you do know that they’re equally far from zero. Either they’re equal (x = y), or one is on the opposite side of zero from the other (x = -y).
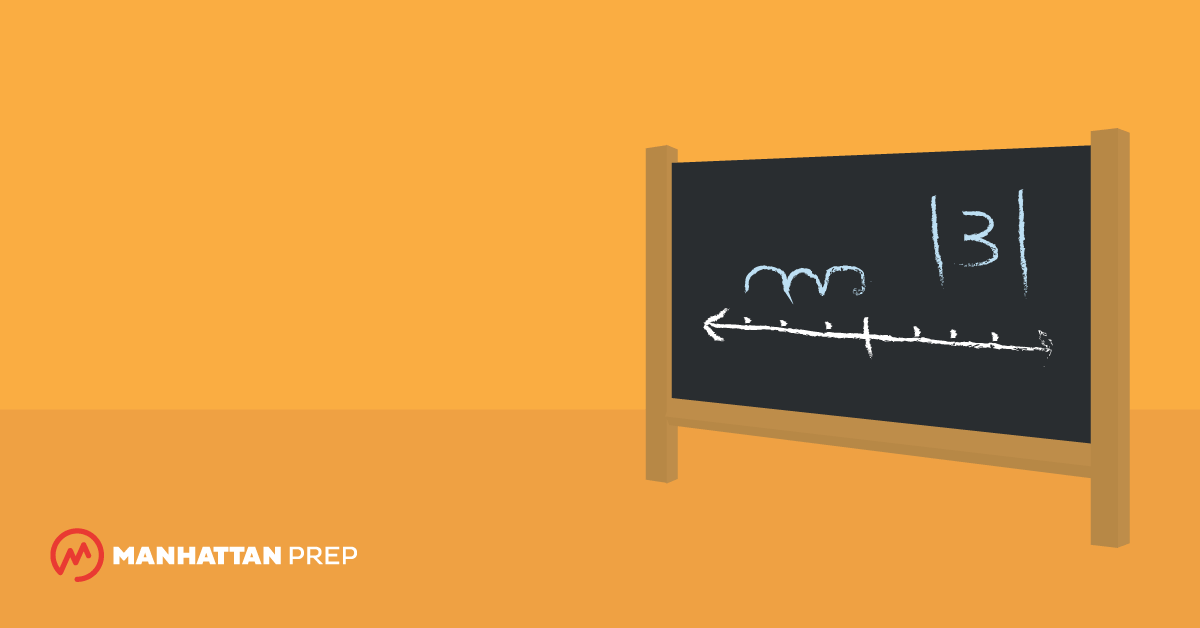
Now, Abby puts a value into the machine, but she won’t even tell you what the machine says. Instead, she just tells you that the answer the machine gave is less than 10. In other words, |x| < 10. What can you say about the value she used?
She could’ve used any value that’s fewer than 10 units away from zero. +5 would’ve worked, and -7 would’ve worked, and 0 would’ve worked. 11, however, wouldn’t work. Neither would -1000. You can actually draw the possibilities out on a number line:
Finally, how about a scenario with both variables and inequalities? You put two variables into the absolute value machine. The machine gives a smaller number in response to the first variable and a larger number in response to the second variable. That is, the machine says that |x| < |y|.
All it’s really saying is that x is closer to zero than y is. That doesn’t tell you anything about whether x and y are positive or negative, and it doesn’t tell you anything about which one of them is greater. x could be closer to zero than y, but also greater:
Or, x could be smaller:
Either way, |x| < |y|.
You don’t have to think about the ‘absolute value machine’ every time you see an absolute value GRE problem. That would be time-consuming and inefficient. However, you should spend some time thinking about it now. If you really grasp where the rules come from, you’ll be less likely to make mistakes when memorizing them and when applying them on test day. You’ll also be better prepared to handle those quirky GRE problems that don’t quite fit. ?
See that “SUBSCRIBE” button in the top right corner? Click on it to receive all our GRE blog updates straight to your inbox!
Chelsey Cooley
is a Manhattan Prep instructor based in Seattle, Washington. Chelsey always followed her heart when it came to her education. Luckily, her heart led her straight to the perfect background for GMAT and GRE teaching: she has undergraduate degrees in mathematics and history, a master’s degree in linguistics, a 790 on the GMAT, and a perfect 170Q/170V on the GRE. Check out Chelsey’s upcoming GRE prep offerings here.