GRE Geometry: Three ways to spot similar triangles
 Did you know that you can attend the first session of any of our online or in-person GRE courses absolutely free? We’re not kidding! Check out our upcoming courses here.
Did you know that you can attend the first session of any of our online or in-person GRE courses absolutely free? We’re not kidding! Check out our upcoming courses here.
Certain diagrams appear in tough GRE Geometry problems over and over again. Here are three of our favorites:
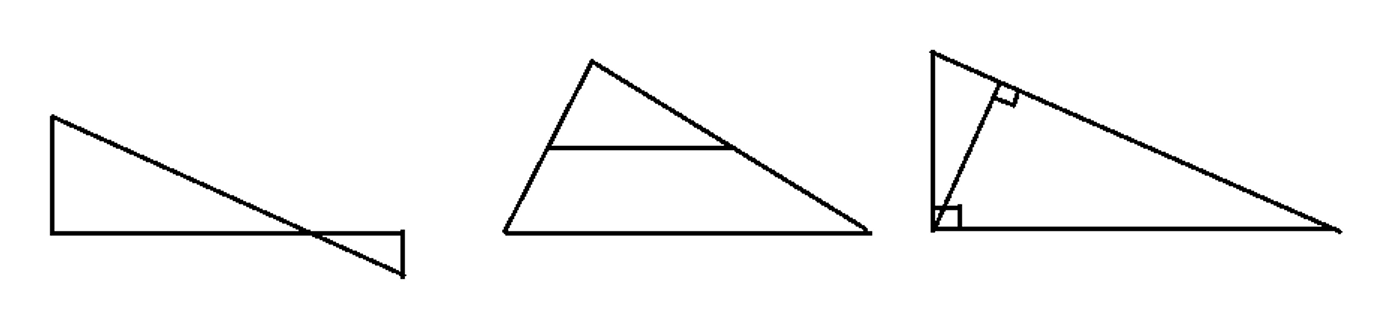
What these three diagrams have in common is that they’re all composed of similar triangles. If you learn to spot them at a glance, you won’t waste time trying to prove that the triangles are similar. You’ll simply recognize that fact, and move on to the next step of the problem.
What are similar triangles, and why are they useful?
In order for two triangles to be similar, they need to have the same angle measurements. They don’t have to be the same size, but if their angles match, they appear to be the same “shape”:
 You don’t need to check all of the angles of a pair of triangles to confirm that they’re similar. Just two pairs of matching angles are enough — can you articulate why that’s the case?
You don’t need to check all of the angles of a pair of triangles to confirm that they’re similar. Just two pairs of matching angles are enough — can you articulate why that’s the case?
If you’ve identified a pair of similar triangles, look for a pair of “matching” sides, and find the ratio between their lengths. That ratio will hold for all three pairs of sides. If the larger triangle has one side that’s twice the length of the smaller triangle’s, that will be true of all three of their sides. That’s what makes similar triangles useful on the GRE: once you identify them, you can work out more information about their side lengths.
So, what about those three diagrams?
The three diagrams above represent the most common ways to disguise a pair of similar triangles.
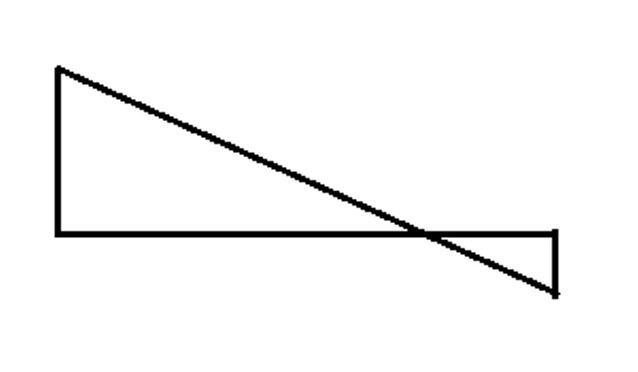
In this diagram, the two vertical sides are parallel. So, the rule of parallel transversals proves that the two angles marked in red are equal:
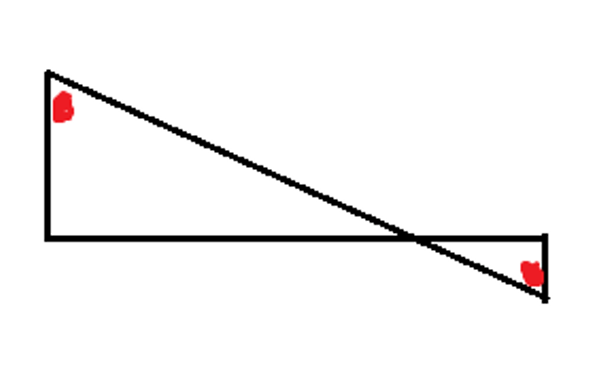
Likewise, there’s a geometry rule that states that if two lines cross each other, the angles created between them are equal. So, the two angles marked in blue are also equal:

With two pairs of matching angles, the two triangles are similar. Memorize the appearance of this diagram, and if you spot it on the GRE, go straight to finding the ratio between side lengths.
The second diagram shows a triangle drawn within another triangle. The two bases are parallel. Again, because of the rule of parallel transversals, the angles marked in the same color are the same:

Try using this pair of similar triangles to solve the following problem from the 5lb. Book of GRE Practice Problems:

Finally, can you prove that all three triangles in the third diagram are similar? Note that they’re all right triangles: that’s critical information. Give it a shot on your own, then check out the diagram below:

Call the smallest angle x, then apply the rule that states that the three angles of a triangle always sum to 180 degrees. Since all of the triangles are right triangles, they each have a 90 degree angle. Use that information to convince yourself that all three triangles have the same angle measurements: x, 90-x, and 90.
How to use this information on the GRE
The toughest thing about similar triangles is spotting them. You can laboriously prove that a pair of triangles is similar by comparing all of their angles, but it’s much easier to be able to recognize the most common diagrams in which these useful triangles appear. If you see a figure on a GRE Geometry problem that looks like one of the three above, immediately start looking for side lengths to compare! Recognize that the triangles in these diagrams will always be similar, and save some time and energy on test day.
Want more guidance from our GRE gurus? You can attend the first session of any of our online or in-person GRE courses absolutely free! We’re not kidding. Check out our upcoming courses here.
Chelsey Cooley is a Manhattan Prep instructor based in Seattle, Washington. Chelsey always followed her heart when it came to her education. Luckily, her heart led her straight to the perfect background for GMAT and GRE teaching: she has undergraduate degrees in mathematics and history, a master’s degree in linguistics, a 790 on the GMAT, and a perfect 170/170 on the GRE. Check out Chelsey’s upcoming GRE prep offerings here.
is a Manhattan Prep instructor based in Seattle, Washington. Chelsey always followed her heart when it came to her education. Luckily, her heart led her straight to the perfect background for GMAT and GRE teaching: she has undergraduate degrees in mathematics and history, a master’s degree in linguistics, a 790 on the GMAT, and a perfect 170/170 on the GRE. Check out Chelsey’s upcoming GRE prep offerings here.
