Did you know that you can attend the first session of any of our online or in-person GRE courses absolutely free? We’re not kidding! Check out our upcoming courses here.

Certain diagrams appear in tough GRE Geometry problems over and over again. Here are three of our favorites:

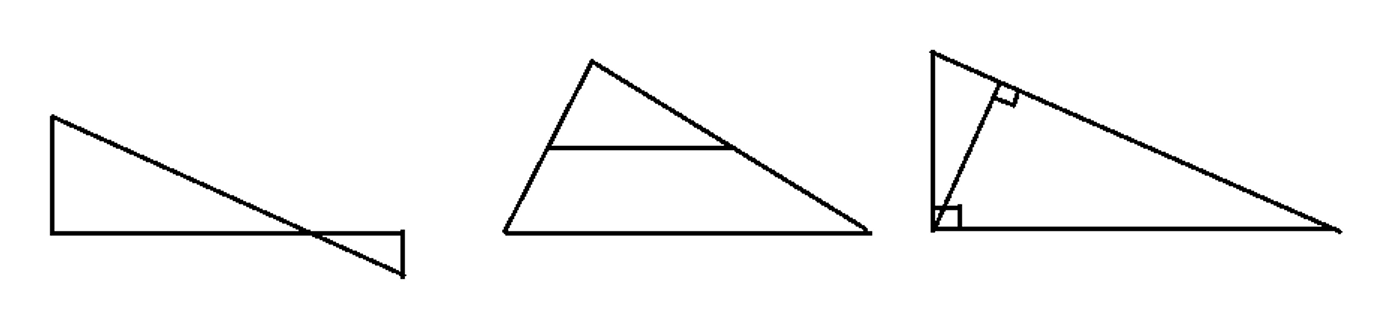
What these three diagrams have in common is that they’re all composed of similar triangles. If you learn to  spot them at a glance, you won’t waste time trying to prove that the triangles are similar.  You’ll simply recognize that fact, and move on to the next step of the problem. Read more

Did you know that you can attend the first session of any of our online or in-person GRE courses absolutely free? We’re not kidding! Check out our upcoming courses here.

Imagine this: you’re at the end of a GRE Quant section, and you have three minutes left. You’ve marked a couple of problems, using the “Good, Bad, and Ugly” technique. Unfortunately, when you look through those problems, there aren’t any that you know you could solve within three minutes. So, what do you do? You’re going to have to guess. Read more

Did you know that you can attend the first session of any of our online or in-person GRE courses absolutely free? We’re not kidding! Check out our upcoming courses here.

Geometry is one of the most polarizing topics on the GRE. If you think it’s great, this article isn’t for you! This set of tips and tricks is for those of us who would rather have a root canal than calculate the length of a hypotenuse.

Check out this Quantitative Comparison problem: Read more

You can attend the first session of any of our online or in-person GRE courses absolutely free. Crazy, right? Check out our upcoming courses here.

Do you remember, when you took exams in high school or college, being allowed to bring a one-page ‘cheat sheet’? I always spent days putting those cheat sheets together in my tiniest handwriting, summarizing an entire semester’s notes on a single page. The funny thing is, by the time I took the exam, I almost never needed to look at the cheat sheet I’d created. After spending all of that time creating it, I had practically memorized my notes. So, even if you can’t bring a GRE Quant Cheat Sheet to the test, you can still benefit from creating one. Synthesizing your notes and thoughts on a single page will give you the ‘big picture’ view of a topic—and will teach you what you do and don’t know. Read more

In one of my recent classes, I told the students “You’ll never know how to answer a geometry question.”  The reaction was fairly predictable: “Why would you say that?!?  That’s so discouraging!!”

Of course, I certainly was NOT trying to discourage them.  I used that statement to illustrate that geometry questions are often a type of quantitative question that can feel immensely frustrating!  You know what shape you have, you know what quantity the question wants, but you have no idea how to solve for that quantity.

This is what I meant when I said you’ll never know how to answer these questions. That “leap” to the correct answer is impossible.  You can’t get to the answer in one step, but that’s all right: you’re not supposed to!

(An important aside: if you’ve read my post regarding calculation v. principle on the GRE, you should be aware that I am discussing the calculation heavy geometry questions in this post.)

The efficient, effective approach to a calculation-based geometry question is NOT to try and jump to the final answer, but instead to simply move to the next “piece”.  For example, let’s say a geometry question gives me an isosceles triangle with two angles equaling x.  I don’t know what x is, and I don’t know how to use it to find the answer to the question.  But I DO know that the third angle is 180-2x.

That’s the game.  Find the next little piece.  And the piece after that.  And the piece after that.  Let’s see an example.

The correct response to this problem is “Bu-whah???  I know nothing about the large circle!”

But you do know the area of the smaller circle.  What piece will that give you?  Ok, you say, area gives me the radius.  A = pi*r^2, so pi = pi*r^2, so r^2 = 1, so r = 1.  Done, and let’s put that in the diagram.
Read more

Harder quant questions combine two different areas of math, and that’s what we’re going to take a look at today.

First, try this problem (© Manhattan Prep) from our Geometry lesson during class 5.

If 2m + 20 > 100, which of the following could be the value of n?
Read more