The 4 Math Strategies Everyone Must Master, part 2
 Last time, we talked about the first 2 of 4 quant strategies that everyone must master: Test Cases and Choose Smart Numbers.
Last time, we talked about the first 2 of 4 quant strategies that everyone must master: Test Cases and Choose Smart Numbers.
Today, we’re going to cover the 3rd and 4th strategies. First up, we have Work Backwards. Let’s try a problem first: open up your Official Guide, 13th edition (OG13), and try problem solving #15 on page 192. (Give yourself about 2 minutes.)
I found this one by popping open my copy of OG13 and looking for a certain characteristic that meant I knew I could use the Work Backwards technique. Can you figure out how I knew, with just a quick glance, that this problem qualified for the Work Backwards strategy? (I’ll tell you at the end of the solution.)
For copyright reasons, I can’t reproduce the entire problem, but here’s a summary: John spends 1/2 his money on fruits and vegetables, 1/3 on meat, and 1/10 on treats from the bakery. He also spends $6 on candy. By the time he’s done, he’s spent all his money. The problem asks how much money he started out with in the first place.
Here are the answer choices:
“(A) $60
“(B) $80
“(C) $90
“(D) $120
“(E) $180”
Work Backwards literally means to start with the answers and do all of the math in the reverse order described in the problem. You’re essentially plugging the answers into the problem to see which one works. This strategy is very closely tied to the first two we discussed last time—except, in this instance, you’re not picking your own numbers. Instead, you’re using the numbers given in the answers.
In general, when using this technique, start with answer (B) or (D), your choice. If one looks like an easier number, start there. If (C) looks a lot easier than (B) or (D), start with (C) instead.
This time, the numbers are all equally “hard,” so start with answer (B). Here’s what you’re going to do:
(B) $80
|
|
F + V (1/2) |
M (1/3) |
B (1/10) |
C $6 |
Add? |
| (B) $80 |
$40 |
…? |
$6 |
Set up a table to calculate each piece. If John starts with $80, then he spends $40 on fruits and vegetables. He spends… wait a second! $80 doesn’t go into 1/3 in a way that would give a dollar-and-cents amount. It would be $26.66666 repeating forever. This can’t be the right answer!
Interesting. Cross off answer (B), and glance at the other answers. They’re all divisible by 3, so we can’t cross off any others for this same reason.
Try answer (D) next.
|
|
F + V (1/2) |
M (1/3) |
B (1/10) |
C $6 |
Add to? |
| (B) $80 |
$40 |
…? |
$6 |
? |
|
| (D) $120 |
$60 |
$40 |
$12 |
$6 |
$118 |
In order for (D) to be the correct answer, the individual calculations would have to add back up to $120, but they don’t. They add up to $118.
Okay, so (D) isn’t the correct answer either. Now what? Think about what you know so far. Answer (D) didn’t work, but the calculations also fell short—$118 wasn’t large enough to reach the starting point. As a result, try a smaller starting point next.
|
|
F + V (1/2) |
M (1/3) |
B (1/10) |
C $6 |
Add? |
| (B) $80 |
$40 |
…? |
$6 |
? |
|
| (D) $120 |
$60 |
$40 |
$12 |
$6 |
$118 |
| (C) $90 |
$45 |
$30 |
$9 |
$6 |
$90 |
It’s a match! The correct answer is (C).
Now, why would you want to do the problem this way, instead of the “straightforward,” normal math way? The textbook math solution on this one involves finding common denominators for three fractions—somewhat annoying but not horribly so. If you dislike manipulating fractions, or know that you’re more likely to make mistakes with that kind of math, then you may prefer to work backwards.
Note, though, that the above problem is a lower-numbered problem. On harder problems, this Work Backwards technique can become far easier than the textbook math. Try PS #203 in OG13. I would far rather Work Backwards on this problem than do the textbook math!
So, have you figured out how to tell, at a glance, that a problem might qualify for this strategy?
It has to do with the form of the answer choices. First, they need to be numeric. Second, the numbers should be what we consider “easy” numbers. These could be integers similar to the ones we saw in the above two problems. They could also be smaller “easy” fractions, such as 1/2, 1/3, 3/2, and so on.
Further, the question should ask about a single variable or unknown. If it asks for x, or for the amount of money that John had to start, then Work Backwards may be a great solution technique. If, on the other hand, the problem asks for x – y, or some other combination of unknowns, then the technique may not work as well.
(Drumroll, please) We’re now up to our fourth, and final, Quant Strategy that Everyone Must Master. Any guesses as to what it is? Try this GMATPrep© problem.

“In the figure above, the radius of the circle with center O is 1 and BC = 1. What is the area of triangular region ABC?
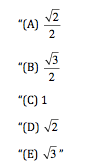
If the radius is 1, then the bottom line (the hypotenuse) of the triangle is 2. If you drop a line from point B to that bottom line, or base, you’ll have a height and can calculate the area of the triangle, since A = (1/2)bh.
You don’t know what that height is, yet, but you do know that it’s smaller than the length of BC. If BC were the height of the triangle, then the area would be A = (1/2)(2)(1) = 1. Because the height is smaller than BC, the area has to be smaller than 1. Eliminate answers (C), (D), and (E).
Now, decide whether you want to go through the effort of figuring out that height, so that you can calculate the precise area, or whether you’re fine with guessing between 2 answer choices. (Remember, unless you’re going for a top score on quant, you only have to answer about 60% of the questions correctly, so a 50/50 guess with about 30 seconds’ worth of work may be your best strategic move at this point on the test!)
The technique we just used to narrow down the answers is one I’m sure you’ve used before: Estimation. Everybody already knows to estimate when the problem asks you for an approximate answer. When else can (and should) you estimate?
Glance at the answers. Notice anything? They can be divided into 3 “categories” of numbers: less than 1, 1, and greater than 1.
Whenever you have a division like this (greater or less than 1, positive or negative, really big vs. really small), then you can estimate to get rid of some answers. In many cases, you can get rid of 3 and sometimes even all 4 wrong answers. Given the annoyingly complicated math that sometimes needs to take place in order to get to the final answer, your best decision just might be to narrow down to 2 answers quickly and then guess.
Want to know how to get to the actual answer for this problem, which is (B)? Take a look at the full solution here.
The 4 Quant Strategies Everyone Must Master
Here’s a summary of our four strategies.
(1) Test Cases.
– Especially useful on Data Sufficiency with variables / unknowns. Pick numbers that fit the constraints given and test the statement. That will give you a particular answer, either a value (on Value DS) or a yes or no (on Yes/No DS). Then test another case, choosing numbers that differ from the first set in a mathematically appropriate way (e.g., positive vs. negative, odd vs. even, integer vs. fraction). If you get an “always” answer (you keep getting the same value or you get always yes or always no), then the statement is sufficient. If you find a different answer (a different value, or a yes plus a no), then that statement is not sufficient.
– Also useful on “theory” Problem Solving questions, particularly ones that ask what must be true or could be true. Test the answers using your own real numbers and cross off any answers that don’t work with the given constraints. Keep testing, using different sets of numbers, till you have only one answer left (or you think you’ve spent too much time).
(2) Choose Smart Numbers.
– Used on Problem Solving questions that don’t require you to find something that must or could be true. In this case, you need to select just one set of numbers to work through the math in the problem, then pick the one answer that works.
– Look for variable expressions (no equals or inequalities signs) in the answer choices. Will also work with fraction or percent answers.
(3) Work Backwards.
– Used on Problem Solving questions with numerical answers. Most useful when the answers are “easy”—small integers, easy fractions, and so on—and the problem asks for a single variable. Instead of selecting your own numbers to try in the problem, use the given answer choices.
– Start with answer (B) or (D). If a choice doesn’t work, cross it off but examine the math to see whether you should try a larger or smaller choice next.
(4) Estimate.
– You’re likely already doing this whenever the problem actually asks you to find an approximate answer, but look for more opportunities to save yourself time and mental energy. When the answers are numerical and either very far apart or split across a “divide” (e.g., greater or less than 0, greater or less than 1), you can often estimate to get rid of 2 or 3 answers, sometimes even all 4 wrong answers.
The biggest takeaway here is very simple: these strategies are just as valid as any textbook math strategies you know, and they also require just as much practice as those textbook strategies. Make these techniques a part of your practice: master how and when to use them, and you will be well on your way to mastering the Quant portion of the GMAT!
Read The 4 Math Strategies Everyone Must Master, Part 1.