Part to Part and Part to Whole Ratios
Did you know that you can attend the first session of any of our online or in-person GMAT courses absolutely free? We’re not kidding! Check out our upcoming courses here.
Take a look at the following problems.
Data Sufficiency: What was Company X’s percentage profit in 2011?
1) The ratio of costs to profits for Company X was 3 to 1 in 2011.
2) Company X’s costs in 2011 were $360,000.
A recipe for punch calls for 4 parts seltzer to one part juice. If John wants to make 5 gallons of punch, how many 8 ounce cans of juice does he need (1 gallon = 128 ounces)?
A) 32
B) 20
C) 16
D) 10
E) 8
Both these problems have something in common. We are given a ratio, but not the specific ratio that would be most useful to our calculation. In the first case, the percentage profit can be calculated at profit/revenue * 100 (remember revenue = profit + costs). In the second case, the ratio that would be most helpful to solving is the ratio of juice to punch, which we could then use to calculate how many ounces of juice we need. It is common for test takers to get stuck at this point not knowing how to get to the ratio needed, but with a little understanding of ratios, it is actually a rather simple task to convert from the given ratios to the ratios that would be most helpful.
Ratios can be divided into part-to-part ratios and part-to-whole ratios. Part-to-part ratios provide the relationship between two distinct groups. For example, the ratio of men to women is 3 to 5, or the solution contains 3 parts water for every 2 parts alcohol.
Part-to-whole ratios provide the relationship between a particular group and the whole populations (including the particular group). For example, 3/5 of the students in the class are girls, or the mixture is 40% rye grass (40% is equivalent to saying 40 of every 100 parts).
If the population for a given problem consists of only two parts, A and B, there are three ratios you can potentially write, one part-to-part ratio and two part-to-whole ratios. Keep in mind that since there are only two parts, the whole is just the sum of these two parts, or A + B:
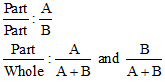
You could write the ratio of B/A, but that is just the reciprocal of the part-to-part ratio already written. In this universe with only two parts, given any one of the three above ratios, you can calculate the other two.
For example, if the ratio of men to women is 3 to 5, we know A=3 and B=5. From here, we can calculate all three ratios (3/5, 3/8, and 5/8). You can also calculate all three ratios if given only one of the part-to-whole ratios. The mixture of flour and sugar is made up of 5/7 flour. In this case, A = 5, A+B=7 making B = 5 -7 = 2.
Recognizing that you can get any of these three ratios given any one is valuable in solving problems because in particular cases, one of the ratios may be more useful to your calculation. Keep in mind that the rules above only apply in cases where there are only two parts. The calculations are not possible in ratios with three or more parts. For example, if a pet store has dogs, cats, and birds, and you are given that 3/7 of the animals are dogs—you cannot calculate the part-to-part ratio of dogs to cats, for example (note: you can calculate the ratio of dogs to cats and birds collectively is 3 to 4).
Let’s take this new knowledge back to our problems from the beginning of this post. In the first problem, statement 1 provides the part-to-part ratio (A = costs, B = profits, A + B = revenues). We are looking for the part-to-whole ratio of B to A+B, so clearly this statement is sufficient. Statement 2 is not sufficient, meaning the answer is A.
For problem 2, we need to calculate how much juice is needed. The total volume of liquid is 640 ounces (5 gallons * 128 ounces per gallon). To calculate the amount of juice, we need the part-to-whole ratio of juice to punch. We are given the part-to-part ratio of seltzer to juice as 4 to 1. This ratio and the formulas provided above enable us to calculate the part-to-whole ratio of juice to punch as 1 to 5. Multiplying this ratio by the total volume of liquid shows that 128 ounces of juice are needed. The final step in solving is dividing 128 by the 8 ounces per can, giving answer C) 16.
This knowledge of ratios is also helpful in working with probability problems. Remember that the definition of probability is successes/total outcomes, a specific part-to-whole ratio. There are only two parts in the case of probability, successes and failures. As such, if you can count the successes and count the failures, you can convert to the correct part-to-whole ratio to calculate the probability:
?
Don’t forget that you can attend the first session of any of our online or in-person GMAT courses absolutely free! Check out our upcoming courses here.
Andrea Pawliczek is a Manhattan Prep instructor based in Denver, Colorado. Andrea is the owner of a perfect 800 GMAT score, a summa cum laude graduate of Emory University with a degree in economics and chemistry, an MBA from Duke’s Fuqua School of Business, and is currently a Ph.D. student in accounting at the University of Colorado – Boulder. Andrea began tutoring during her time at Emory and further honed her teaching skills at Fuqua, where she served as a teaching assistant for accounting and finance courses. Now, she continues to teach financial accounting to CU undergraduates and the GMAT with Manhattan Prep. Check out her upcoming GMAT courses here.