As dedicated readers of this blog may have guessed, this is a follow up to my earlier post When is it Time to Guess on Quant? Timing troubles are not, however, exclusive to the Quant section, so in this piece I’ll talk about some common scenarios that bedevil students on the Verbal section.

As with Quant, not all guesses are created equal. The earlier you decide to guess, the more likely that you will make a random guess. If, on the other hand, you’re far enough into the question that you’ve eliminated 2-3 answer choices, then you’ll be making an educated guess.

One immediate difference between guessing on Quant and Verbal is that guessing strategy is essentially identical for both Problem Solving and Data Sufficiency questions. Each of the Verbal question types, on the other hand, has less in common. That being said, there are a lot of parallels in guessing strategy among the three types.

No matter the question, there are really three distinct stages at which it becomes a better idea to guess than to keep going. I’ll briefly describe each stage, then show how it connects to each of the Verbal question types.

Stage 1: No Clear Starting Point

As a general rule, if you haven’t really made progress on a question after 30 seconds or so, it’s usually a good idea to just make a random guess and save your energy for a question you’re more comfortable with.

Reading Comprehension Stage 1: I don’t know where in the passage to look.

The great thing about Reading Comprehension (or at least its saving grace) is that the correct answer has to have support in the passage. With the vast majority of RC questions, as long as you can find and reread the relevant portion of the passage, you can find an answer choice that will match what you read. In fact, you should be able to answer to come up with your own answer to most RC questions before you even look at the answer choices.

Many questions provide good clues as to where in the passage to look for the answer (seriously – a surprising amount of questions are very helpful in that regard). Things get much tougher when they don’t. So here’s your first big clue that it may be time to guess. If you’ve read the question, and you’ve skimmed through the passage looking for an answer, and you still don’t feel like you found what the question was asking about, it’s time to guess.

At this point, you could guess randomly, but I would recommend taking one quick pass through the answer choices. If any choice contradicts your understanding of the passage, eliminate it. After you’ve each answer once, pick from the remaining.

Sentence Correction Stage 1: I don’t understand the sentence and the underline is long.

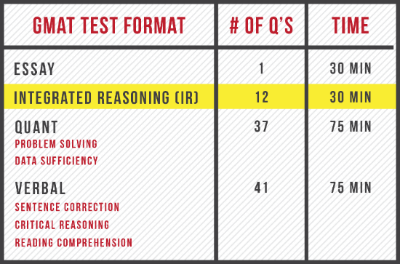
On the Verbal section, you have to answer 41 questions in 75 minutes, which is less than 2 minutes per question. Critical Reasoning and Reading Comprehension are naturally time-consuming, so that time is going to have be saved largely on Sentence Correction. Remember that you only have an average of 1 minute and 20 seconds to answer these things.

If you’re struggling to even understand what the sentence is saying, then it will almost certainly take too long to properly analyze the answer choices, especially if the underline is long. No need to fight through the pain. Just take a quick scan through the answer choices and pick one that doesn’t sound immediately wrong.

Critical Reasoning Stage 1: I don’t understand what the argument is saying.

To my mind, good process on Critical Reasoning questions means being in control the whole way through the process. The worst situation to be in is one in which you’re hoping that the answer choices will help you make sense of the argument. Four out of the five answer choices are actively trying to trick you, and the GMAT has gotten pretty good at tricking people over the years. By the time you get to the answer choices, you need to understand the argument well enough to effectively evaluate each choice.

Consequently, if you’ve read the argument two or three times, and still can’t articulate to yourself the link between the premises and the conclusion, you shouldn’t waste time with the answer choices.

  Read more

Did you know that you can attend the first session of any of our online or in-person GMAT courses absolutely free? We’re not kidding! Check out our upcoming courses here.

I first wrote about this topic back in 2010. I’ve seen so many people lately on the forums who are going for a 750 or 760 score or higher that I decided to revive the conversation. Read more

The GMAT quant section has many faces – there are a number of content areas, and it is best to try to master as many of them as you can before test day. It is important, however, that you not compartmentalize too much. In many of the harder questions in fact, two or more topics often show up together. You can easily find quadratics in a consecutive integer question, coordinate geometry in a probability question, number properties in a function question, for example. One common intersection of two topics that I find surprises many students is that of geometry and algebra. Many people expect a geometry question to be about marking up diagrams with values or tick marks to show equality and/or applying properties and formulas to calculate or solve. While these are no doubt important skill sets in geometry, don’t forget to pull out one of the most important skills from your  GMAT tool bag – the almighty variable! x’s and y’s have a welcomed home in many a geometry question, though you might find that you are the one who has to take the initiative to put them there!

Take a look at this data sufficiency question from GMATPrep®

In the figure shown, the measure of PRS is how many degrees greater than the measure of PQR?

(1) The measure of QPR is 30 degrees.

(2) The sum of the measures of  PQR and PRQ is 150 degrees.

How did you do? Don’t feel bad if you’re a little lost on this one. This is a difficult question, though you’ll see that with the right moves it is quite doable. At the end of this discussion, you’ll even see how you could put up a good guess on this one.

As is so often the case in a data sufficiency question, the right moves here start with the stem – in rephrasing the question. Unfortunately the stem doesn’t appear to provide us with a lot of given information. As indicated in the picture, you have a 90 degree angle at  PQR and that seems like all that you are given, but it’s not! There are some other inherent RELATIONSHIPS, ones that are implied by the picture. For example PRS and PRQ sum to 180 degrees. The problem, however, is how do you CAPTURE THOSE RELATIONSHIPS? The answer is simple – you capture those relationships the way you always capture relationships in math when the relationship is between two unknown quantities – you use variables!

But where should you put the variables and how many variables should you use?  This last question is one that you’ll likely find yourself pondering  a number of times on the GMAT. Some believe the answer to be a matter of taste. My thoughts are always use as few variables as possible. If you can capture all of the relationships that you want to capture with one variable, great. If you need two variables, so be it. The use of three or more variables would be rather uncommon in a geometry question, though you could easily see that in a word problem.  Keep one thing in mind when assigning variables: the more variables you use, usually the more equations you will need to write in order to solve.

As for the first question above about where to place the variables, you can take a closer look in this question at what they are asking and use that as a guide. They ask for the   (degree) difference between PRS and PQR. Since PRS is in the question, start by labeling PRS as x. Since PRS and  PRQ sum to 180 degrees, you can also label PRQ as (180 – x) and  RPS as (180 – x – 90) or (90 – x).

Can you continue to label the other angles in triangle PRQ in terms of x or is it now time to place a second variable, y? Since you still have two other unknown quantities in that triangle, it’s in fact time for that y. The logical place of where to put it is on  PQR since that is also part of the actual question. The temptation is to stop there – DON’T! Continue to label the final angle of the triangle, QPR, using your newfound companions, x and y. QPR can be labeled as [180 – y – (180 – x)] or (x – y). Now all of the angles in the triangle are labeled and you are poised and ready to craft an algebraic equation/expression to capture any other relationships that might come your way.

Before you rush off to the statements, however, there is one last step. Formulate what the question is really asking in terms of x and y. The question rephrases to “What is the value of x – y?”

Now you can finally head to the statements. Oh the joy of a fully dissected data sufficiency stem – 90% of the work has already been done!

Statement (1) tells you that the measure of QPR is 30 degrees. Using your x – y expression from the newly labeled diagram as the value of  QPR, you can jot down the equation x – y = 30. Mission accomplished! The statement is sufficient to answer the question “what is the value of x – y?”

Statement (2) indirectly provides the same information as statement (1). If the two other angles of triangle PQR sum to 150 degrees, then QPR is 30 degrees, so the statement is sufficient as well. If you somehow missed this inference and instead directly pulled from the diagram y + (180 – x) and set that equal to 150, you’d come to the same conclusion. Either way the algebra saves the day!

The answer to the question is D, EACH statement ALONE is sufficient to answer the question asked.

NOTE here that from a strategic guessing point of view, noticing that statements (1) and (2) essentially provide the same information allows you to eliminate answer choices A, B and C: A and B because how could it be one and not the other if they are the same, and C because there is nothing gained by combining them if they provide exactly the same information.

The takeaways from this question are as follows:

(1) When a geometry question has you staring at the diagram, uncertain of how to proceed in marking things up or capturing relationships that you know exist – use variables! Those variables will help you move through the relationships just as actual values would.

(2) In data sufficiency geometry questions, when possible represent the question in algebraic form so the target becomes clear and so that the rules of algebra are there to help you assess sufficiency.

(3) Once you have assigned a variable, continue to label as much of the diagram in terms of that variable. If you need a second variable to fully label the diagram, use it. If you can get away with just one variable and still accomplish the mission, do so.

Most GMAT test-takers know that they need to develop clear strategies when it comes to different types of word problems, and most of those involve either muscling your way through the problem with some kind of practical approach (picking numbers, visualizing, back-solving, logical reasoning) or writing out algebraic equations and solving.  There are of course pluses and minuses to all of the approaches and those need to be weighed by each person on an individual basis. What few realize, however, is that geometry questions can also demonstrate that level of complexity and thus can often also be solved with the tools of algebra. When actual values are few and far between, don’t hesitate to pull out an “x” (and possibly also a “y”) and see what kind of equations/expressions you can cook up.

For more practice in “algebrating” a geometry question, please see OG 13^th DS 79 and Quant Supplement 2^nd editions PS 157, 162 and DS 60, 114 and 123.

Integrated Reasoning, the newest addition to the GMAT, was added to the GMAT in response to real skills employers are looking for in new hires – namely, the ability to analyze information presented in multiple ways – in order to succeed in today’s data-driven workplace. Sounds tough, right? The good news is that Integrated Reasoning can be learned.

And we’ve created a new tool to teach it—available for free for a limited time only!

The complete GMAT INTERACT platform (coming in June!) will teach every section of the GMAT, but you can get started on the IR section right now, for free. It won’t be available for free forever, though, so be sure to sign up before it’s too late!

Are you ready to learn Integrated Reasoning? Try GMAT INTERACT for Integrated Reasoning for free here.

//youtu.be/fi1Do93UizU

Please join us for an exciting, online open house to learn about the rewarding teaching opportunities with Manhattan Prep.

We are seeking expert teachers throughout the US who have proven their mastery of the GMAT, GRE or LSAT and who can engage students of all ability levels. Our instructors teach in classroom and one-on-one settings, both in-person and online. We provide extensive, paid training and a full suite of print and digital instructional materials. Moreover, we encourage the development and expression of unique teaching styles..

All Manhattan Prep instructors earn $100/hour for teaching and tutoring – up to four times the industry standard.  These are part-time positions with flexible hours. Many of our instructors maintain full-time positions, engage in entrepreneurial endeavors, or pursue advanced degrees concurrently while teaching for Manhattan Prep.  (To learn more about our exceptional instructors, read their bios or view this short video.

Learn about how to transform your passion for teaching into a lucrative and fulfilling part-time career by joining us for this Online Open House event!

To attend this free event, please select from one of the following online events and follow the on-screen instructions:

About Manhattan Prep

Manhattan Prep is a premier test-preparation company serving students and young professionals studying for the GMAT (business school), LSAT (law school), GRE (master’s and PhD programs), and SAT (undergraduate programs).  We are the leading provider of GMAT prep in the world.

Manhattan Prep conducts in-person classes and private instruction across the United States, Canada, and England.  Our online courses are available worldwide, and our acclaimed Strategy Guides are available at Barnes & Noble and Amazon.  In addition, Manhattan Prep serves an impressive roster of corporate clients, including many Fortune 500 companies.  For more information, visit www.manhattanprep.com.

A while back, we talked about the 4 GMAT math strategies that everyone needs to master. Today, I’ve got some additional practice for you with regard to one of those strategies: Testing Cases.

Try this GMATPrep® problem:

* ” If xy + z = x(y + z), which of the following must be true?

“(A) x = 0 and z = 0

“(B) x = 1 and y = 1

“(C) y = 1 and z = 0

“(D) x = 1 or y = 0

“(E) x = 1 or z = 0

How did it go?

This question is called a “theory” question: there are just variables, no real numbers, and the answer depends on some characteristic of a category of numbers, not a specific number or set of numbers. Problem solving theory questions also usually ask what must or could be true (or what must not be true). When we have these kinds of questions, we can use theory to solve—but that can get very confusing very quickly. Testing real numbers to “prove” the theory to yourself will make the work easier.

The question stem contains a given equation:

xy + z = x(y + z)

Whenever the problem gives you a complicated equation, make your life easier: try to simplify the equation before you do any more work.

xy + z = x(y + z)

xy + z = xy + xz

z =  xz

Very interesting! The y term subtracts completely out of the equation. What is the significance of that piece of info?

Nothing absolutely has to be true about the variable y. Glance at your answers. You can cross off (B), (C), and (D) right now!

Next, notice something. I stopped at z = xz. I didn’t divide both sides by z. Why?

In general, never divide by a variable unless you know that the variable does not equal zero. Dividing by zero is an “illegal” move in algebra—and it will cause you to lose a possible solution to the equation, increasing your chances of answering the problem incorrectly.

The best way to finish off this problem is to test possible cases. Notice a couple of things about the answers. First, they give you very specific possibilities to test; you don’t even have to come up with your own numbers to try. Second, answer (A) says that both pieces must be true (“and”) while answer (E) says “or.” Keep that in mind while working through the rest of the problem.

z =  xz

Let’s see. z = 0 would make this equation true, so that is one possibility. This shows up in both remaining answers.

If x = 0, then the right-hand side would become 0. In that case, z would also have to be 0 in order for the equation to be true. That matches answer (A).

If x = 1, then it doesn’t matter what z is; the equation will still be true. That matches answer (E).

Wait a second—what’s going on? Both answers can’t be correct.

Be careful about how you test cases. The question asks what MUST be true. Go back to the starting point that worked for both answers: z = 0.

It’s true that, for example, 0 = (3)(0).

Does z always have to equal 0? Can you come up with a case where z does not equal 0 but the equation is still true?

Try 2 = (1)(2). In this case, z = 2 and x = 1, and the equation is true. Here’s the key to the “and” vs. “or” language. If z = 0, then the equation is always 0 = 0, but if not, then x must be 1; in that case, the equation is z = z. In other words, either x = 1 OR z = 0.

The correct answer is (E).

The above reasoning also proves why answer (A) could be true but doesn’t always have to be true. If both variables are 0, then the equation works, but other combinations are also possible, such as z = 2 and x = 1.

Key Takeaways: Test Cases on Theory Problems

(1) If you didn’t simplify the original equation, and so didn’t know that y didn’t matter, then you still could’ve tested real numbers to narrow down the answers, but it would’ve taken longer. Whenever possible, simplify the given information to make your work easier.

(2) Must Be True problems are usually theory problems. Test some real numbers to help yourself understand the theory and knock out answers. Where possible, use the answer choices to help you decide what to test.

(3) Be careful about how you test those cases! On a must be true question, some or all of the wrong answers could be true some of the time; you’ll need to figure out how to test the cases in such a way that you figure out what must be true all the time, not just what could be true.

* GMATPrep® questions courtesy of the Graduate Management Admissions Council. Usage of this question does not imply endorsement by GMAC.

Have you heard of the C-Trap? I’m not going to tell you what it is yet. Try this problem from GMATPrep® first and see whether you can avoid it

* “In a certain year, the difference between Mary’s and Jim’s annual salaries was twice the difference between Mary’s and Kate’s annual salaries. If Mary’s annual salary was the highest of the 3 people, what was the average (arithmetic mean) annual salary of the 3 people that year?

“(1) Jim’s annual salary was $30,000 that year.

“(2) Kate’s annual salary was $40,000 that year.”

I’m going to do something I normally never do at this point in an article: I’m going to tell you the correct answer. I’m not going to type the letter, though, so that your eye won’t inadvertently catch it while you’re still working on the problem. The correct answer is the second of the five data sufficiency answer choices.

How did you do? Did you pick that one? Or did you pick the trap answer, the third one?

Here’s where the C-Trap gets its name: on some questions, using the two statements together will be sufficient to answer the question. The trap is that using just one statement alone will also get you there—so you can’t pick answer (C), which says that neither statement alone works.

In the trickiest C-Traps, the two statements look almost the same (as they do in this problem), and the first one doesn’t work. You’re predisposed, then, to assume that the second statement, which seemingly supplies the “same” kind of information, also won’t work. Therefore, you don’t vet the second statement thoroughly enough before dismissing it—and you’ve just fallen into the trap.

How can you dig yourself out? First of all, just because two statements look similar, don’t assume that they either both work or both don’t. The test writers are really good at setting traps, so assume nothing.
Read more

Recently, we talked about how to create Official Guide (OG) problem sets in order to practice for the test. I have one more component to add: track your work and analyze your results to help you prioritize your studies.

In the first half of this article, we talked about making problem sets from the roughly 1,500 problems that can be found in the three main OG books. These problems are generally regarded as the gold standard for GMAT study, but how do you keep track of your progress across so many different problems?

The best tool out there (okay, I’m biased) is our GMAT Navigator program, though you can also build your own tracking tool in Excel, if you prefer. I’ll talk about how to get the most out of Navigator, but I’ll also address what to include if you decide to build your own Excel tracker.

(Note: GMAT Navigator used to be called OG Archer. If you used OG Archer in the past, Navigator brings you all of that same functionality—it just has a new name.)

What is GMAT Navigator?

Navigator contains entries for every one of the problems in the OG13, Quant Supplement, and Verbal Supplement books. In fact, you can even look up problems from OG12. You can time yourself while you answer the question, input your answer, review written and video solutions, get statistics based on your performance, and more.

Everyone can access a free version of Navigator. Students in our courses or guided-self study programs have access to the full version of the program, which includes explanations for hundreds of the problems.

How Does Navigator Work?

First, have your OG books handy. The one thing the program does not contain is the full text of problems. (Copyright rules prevent this, unfortunately.)

When you sign on to Navigator, you’ll be presented with a quick tutorial showing you what’s included in the program and how to use it. Take about 10 minutes to browse through the instructions and get oriented.

When you reach the main page, your first task is to decide whether you want to be in Browse mode or Practice mode.

Practice mode is the default mode; you’ll spend most of your time in this mode. You’ll see an entry for the problem along with various tools (more on this below).

Browse mode will immediately show you the correct answer and the explanation. You might use this mode after finishing a set of questions, when you want to browse through the answers. Don’t reveal the answers and explanations before you’ve tried the problem yourself!

Here’s what you can do in Practice mode:
Read more

Some Data Sufficiency questions present you with scenarios: stories that could play out in various complicated ways, depending on the statements. How do you get through these with a minimum of time and fuss?

Try the below problem. (Copyright: me! I was inspired by an OG problem; I’ll tell you which one at the end.)

* “During a week-long sale at a car dealership, the most number of cars sold on  any one day was 12. If at least 2 cars were sold each day, was the average daily number of cars sold during that week more than 6?

“(1) During that week, the second smallest number of cars sold on any one day was 4.

“(2) During that week, the median number of cars sold was 10.”

First, do you see why I described this as a “scenario” problem? All these different days… and some number of cars sold each day… and then they (I!) toss in average and median… and to top it all off, the problem asks for a range (more than 6). Sigh.

Okay, what do we do with this thing?

Because it’s Data Sufficiency, start by establishing the givens. Because it’s a scenario, Draw It Out.

Let’s see. The “highest” day was 12, but it doesn’t say which day of the week that was. So how can you draw this out?

Neither statement provides information about a specific day of the week, either. Rather, they provide information about the least number of sales and the median number of sales.

The use of median is interesting. How do you normally organize numbers when you’re dealing with median?

Bingo! Try organizing the number of sales from smallest to largest. Draw out 7 slots (one for each day) and add the information given in the question stem:

Now, what about that question? It asks not for the average, but whether the average number of daily sales for the week is more than 6. Does that give you any ideas for an approach to take?

Because it’s a yes/no question, you want to try to “prove” both yes and no for each statement. If you can show that a statement will give you both a yes and a no, then you know that statement is not sufficient. Try this out with statement 1

(1) During that week, the least number of cars sold on any one day was 4.

Draw out a version of the scenario that includes statement (1):

Can you find a way to make the average less than 6? Keep the first day at 2 and make the other days as small as possible:

The sum of the numbers is 34. The average is 34 / 7 = a little smaller than 5.

Can you also make the average greater than 6? Try making all the numbers as big as possible:

(Note: if you’re not sure whether the smallest day could be 4—the wording is a little weird—err on the cautious side and make it 3.)

You may be able to eyeball that and tell it will be greater than 6. If not, calculate: the sum is 67, so the average is just under 10.

Statement (1) is not sufficient because the average might be greater than or less than 6. Cross off answers (A) and (D).

Now, move to statement (2):

(2) During that week, the median number of cars sold was 10.

Again, draw out the scenario (using only the second statement this time!).

Can you make the average less than 6? Test the smallest numbers you can. The three lowest days could each be 2. Then, the next three days could each be 10.

The sum is 6 + 30 + 12 = 48. The average is 48 / 7 = just under 7, but bigger than 6. The numbers cannot be made any smaller—you have to have a minimum of 2 a day. Once you hit the median of 10 in the middle slot, you have to have something greater than or equal to the median for the remaining slots to the right.

The smallest possible average is still bigger than 6, so this statement is sufficient to answer the question. The correct answer is (B).

Oh, and the OG question is DS #121 from OG13. If you think you’ve got the concept, test yourself on the OG problem.

Key Takeaway: Draw Out Scenarios

(1) Sometimes, these scenarios are so elaborate that people are paralyzed. Pretend your boss just asked you to figure this out. What would you do? You’d just start drawing out possibilities till you figured it out.

(2) On Yes/No DS questions, try to get a Yes answer and a No answer. As soon as you do that, you can label the statement Not Sufficient and move on.

(3) After a while, you might have to go back to your boss and say, “Sorry, I can’t figure this out.” (Translation: you might have to give up and guess.) There isn’t a fantastic way to guess on this one, though I probably wouldn’t guess (E). The statements don’t look obviously helpful at first glance… which means probably at least one of them is!

Did you know that you can attend the first session of any of our online or in-person GMAT courses absolutely free? We’re not kidding! Check out our upcoming courses here.

I’m reviving an old article I first wrote five years ago (time flies!) because the topic is so important. I hope that no one ever again experiences a significant GMAT score drop on the real test (or even a practice one!), but the reality is that this does happen. The big question: now what? Read more